Learn to Count Your Days.

By Brent Pritchard

Prior to when teaching and a desire to expand my influence outside of Corporate America won the day, I worked as a commercial mortgage loan officer for the real estate arm of a global life insurance company. One of my favorite mortgage bankers and someone I’m proud to call a friend to this day is Peter Dailey. (In case you’re wondering, this isn’t his real name.) Speaking of days, let’s discuss day-count conventions.

Here’s an excerpt from my book Would Your Boomerang Return? What Birds, Hurdlers, and Boomerangs Can Teach Us About the Time Value of Money (2023):

The most common day-count conventions are 30/360, Actual/360, 30/365, Actual/365, and Actual/Actual. For each day-count convention, the last number or word represents the agreed-upon number of days in a given year, and the first number or word represents the assumed number of days in a given month. The day-count convention 30/360 is also known as the “banker’s year.”

The “banker’s year” assumes that there are 360 days in a year and 30 days in each month. The 30/360 day-count convention makes the math easy. Not all mortgage “bankers” are as good with math as Peter and need some help!

A mortgage loan is an example of a negotiated agreement, and the borrower and lender agree that for the purpose of calculating the simple daily interest rate, the assumption will be that there are 360 days in a year, and that for the purpose of calculating the simple accrued interest, it will be assumed that there are 30 days in each month.

In the inc shorthand for most mortgage loans, “c” would be 12 and the “n” would be 1. But this is really a 30/360 “banker’s year” day-count convention in disguise, since you could divide the simple annual interest rate by 360 (/360) and then multiply by 30 to ultimately get the true monthly interest rate: the “c” isn’t 360 because the loan document doesn’t call for daily compounding or 360 compounding periods within the time span of the simple interest rate.

Now let’s run the numbers using the inc shorthand “0.005, 1, 12.” If this were a mortgage loan that required monthly debt service payments with interest calculated and accrued based on a 30/360 day-count convention, you could back into the simple annual interest rate of 6% in an unconventional way using the day-count convention:

Illustrations by Scott Alberts. Copyright 2022 Brent Pritchard. All rights reserved.

You’ve been working with a “banker’s year” day-count convention all along, even if you didn’t realize it.

Today’s the day to learn more about day-count conventions, if you don’t have a handle on them already, because a finance professional who doesn’t know about day-count conventions is a finance professional whose days in the industry may be numbered.

Who is the first person to educate you on this topic of day-count conventions? (If not your finance instructor, then what’s up with that?!)

---

Brent Pritchard is an author and college finance lecturer with over two decades of industry experience and cofounder of Boxholm Press, LLC, a family-owned-and-operated publishing company providing educational content, products, and services. He pioneers an innovative and approachable new way of learning and teaching the Time Value of Money as well as thought leadership in other business topics. His most recent book is Would Your Boomerang Return? You can contact him on his website here.

The Only Rate That Will Fly!

By Brent Pritchard

In Mrs. Pritchard’s second-grade classroom, one of the favorite lessons is the butterfly lesson. This is the one where students get to see the little caterpillar grow before forming a chrysalis and then turning into a monarch butterfly that will fly away.

The second part to this lesson is the finance version. In my book Would Your Boomerang Return? I write about matching the hatch, a concept that comes from fly fishing, to describe the requirement to correspond or match the time span in between periods or payments (on the timeline) and the time span of the true investment yield. Without the match there’s no way to discount or compound. For those who have read the book or followed this blog, this is TVM Rule #2.

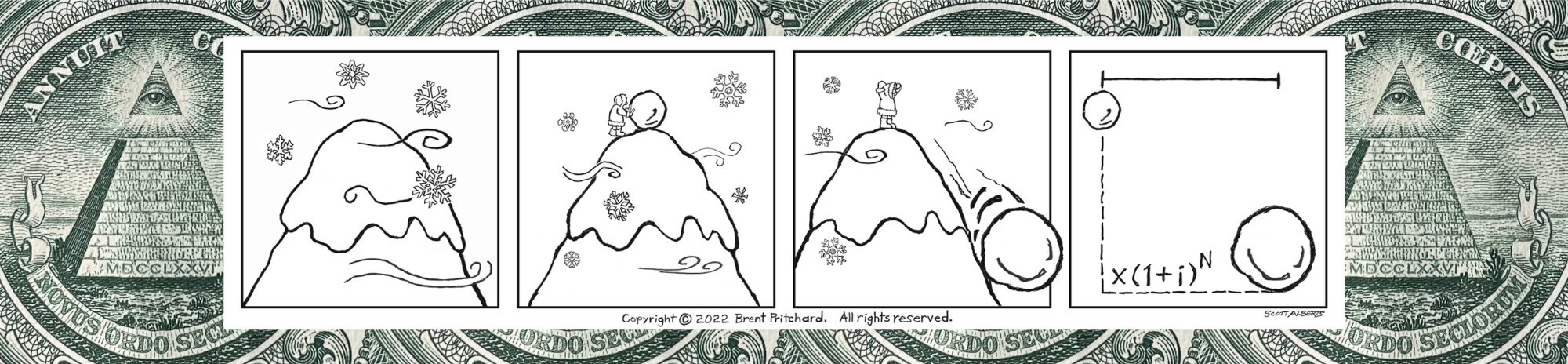
The best way I’ve found to teach students how to manipulate the investment yield starts with the building block True Investment Yield equation and then gets them to call the individual yields or rates by name. Let’s take a look at this building block Time Value of Money equation:

Illustration by Scott Alberts. Copyright 2022 Brent Pritchard. All rights reserved.

Ignoring the boomerang for a moment, use your imagination to see a caterpillar in the “s,” which is a simple investment yield that doesn’t consider the effects of compounding. This is what I refer to as the “caterpillar rate.” Let’s assume our caterpillar rate was a 1.50% simple quarterly investment yield. We’ve called it by name just like we can name a caterpillar.

Here’s where most people stop calling the investment yield by name, which is the main reason why I think people struggle manipulating investment yields. Keep that in mind as you calculate the “chrysalis rate.” I can see the shape of a chrysalis in the parentheses around “s” divided by “c.” Can you? If the 1.50% simple quarterly investment yield compounded monthly, there are 3 compounding periods within the time span of the simple investment yield. So, you would divide .015 by 3. But that’s not all. Let’s call this simple investment yield by name. The chrysalis rate is a 0.50% simple monthly investment yield.

We’re left with the “butterfly rate,” which is the only investment yield that will fly when it comes to applying the Time Value of Money. Let’s assume the time span in between periods or payments (on the timeline) is one year. The only rate that will fly is a true annual investment yield. Since our chrysalis rate is stated in monthly terms, how many months are in a year? That’s the value you need for “n.” And after subtracting one we call it by name. The butterfly rate is a 6.1678% true annual investment yield.

And there are times when a caterpillar is on a fish’s menu…. You can’t make this stuff up.

How do you feel about your ability to manipulate the investment yield? (Without it you can’t apply the Mathematics of Finance.)

---

Brent Pritchard is an author and college finance lecturer with over two decades of industry experience and cofounder of Boxholm Press, LLC, a family-owned-and-operated publishing company providing educational content, products, and services. He pioneers an innovative and approachable new way of learning and teaching the Time Value of Money as well as thought leadership in other business topics. His most recent book is Would Your Boomerang Return? You can contact him on his website here.

The Perfect Timeline.

By Brent Pritchard

Recently, our daughters were surprised to find out that their dad was awarded an art scholarship right out of high school. I’ve always had a creative side.

In college, my fellow art classmates used to like to razz me about selling out as I packed up my supplies and prepared to walk to my business classes. It was all in good fun. Looking back, I’ve always looked for balance in life in one way or another. This was just that.

In your finance classes, you might hear an instructor say something along the lines of, “When in doubt, draw a timeline.”

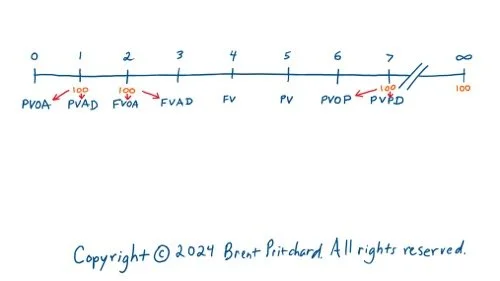
Now there’s no doubt in my mind that what follows just might be The Perfect Timeline. I’m not referring to straight lines or symmetry; rather, an illustration that can help business students see how the Building Block Time Value of Money equations interact with the timeline and specific payments. Behold, The Perfect Timeline:

There are a few things to point out. First, note how the tip of the arrow resembles the letter V. This reminds us where the (Present or Future) Value is under the timeline in relation to the first or last payment for an annuity and the first payment for a perpetuity. The abbreviations are as follows:

• Present Value of an Ordinary Annuity (PVOA);

• Present Value of an Annuity Due (PVAD);

• Future Value of an Ordinary Annuity (FVOA);

• Future Value of an Annuity Due (FVAD);

• Present Value of an Ordinary Perpetuity (PVOP);

• Present Value of a Perpetuity Due (PVPD);

• And last but not least, Future Value (FV) and Present Value (PV).

The Perfect Timeline requires that you have a certain level of comprehension with regard to the Building Block Time Value of Money equations and how a lump-sum or single payment is different from an even series, etc.

Assuming a 5% true investment yield, which is what some might call a compound interest rate, can you find the following values:

• PVOA: 185.94 (euro)

• PVAD: 195.24 (euro)

• FVOA: 205 (euro)

• FVAD: 215.25 (euro)

• PVOP: 2,000 (euro)

• PVPD: 2,100 (euro)

• FV of the FVAD: 226.01 (euro)

• PV of the PVPD: 1,904.76 (euro)

Now consider the following:

• The timeline is silent with regard to the time span in between periods or payments. This was on purpose to illustrate a point. Does the space in between each time period marker represent one year, six months, a quarter, a month, a week? This is an important question, because you can’t compound or discount moneys below the timeline unless you have an “i” that matches “n.” Enter the last of the Building Block Time Value of Money equations: the True Investment Yield equation.

• Notice how we could add a “1” to each time period marker and consider a different range of time.

• If you’re running the numbers using the building block Time Value of Money equations for an annuity, pay attention to the relationship between “N” and “PMT.”

• Did you catch that for the FVOA the second in the series of two even payments doesn’t earn a return on investment.

• For those fluent with the building block Time Value of Money equations for an annuity or perpetuity, see how the add-on expression, (1 + i), just moves the PVOA and FVOA and PVOP one period into the future to calculate the PVAD and FVAD and PVPD, respectively. As it relates to the future value associated with the even series with two even payments that last payment gets its turn to earn a return on investment, and the other payment gets to “work” for one more period.

• Notice how the PVOA is as of one period before the first even payment in the series.

• See how FVAD can sub-in for PV in the Building Block Future Value equation. Sooner or later, the future becomes the present!

• If you’re doing the work and putting in the reps, first I congratulate you for getting this far! Now, did you notice how for the PVPD or PVOP the value of the investment is maintained at $2,000. For the PVPD, do you understand that the 100 (euro) payment happens right way, thus reducing the amount of money invested from 2,100 to 2,000 (euro). And finally, for the PVOP, do you understand that the 2,000 (euro) investment earns a return on investment for one period before the first payment in the series is made.

• Notice how the PV doesn’t need to be as of (t)o(day) or (n)o(w); i.e., time period marker zero on the timeline.

There you have it, The Perfect Timeline. I don’t think our illustrator is going to be worried about not hearing from us again! Forget about it!

What do you think?

---

Brent Pritchard is an author and college finance lecturer with over two decades of industry experience and cofounder of Boxholm Press, LLC, a family-owned-and-operated publishing company providing educational content, products, and services. He pioneers an innovative and approachable new way of learning and teaching the Time Value of Money as well as thought leadership in other business topics. His most recent book is Would Your Boomerang Return? You can contact him on his website here.